How to Multiply on the Abacus with Single Digit Multiplier?
Multiplication on the abacus is simple, fast, and efficient. The pre-requisites for learning how to multiply on the abacus are two fold. First students must know how to add on the abacus since multiplication is a series of additions. If you don’t know addition on the abacus or are new to abacus math please take a look at our beginning abacus lessons before proceeding here. Secondly students must memorize their 9×9 math facts with the goal of fast recall. The faster a student can recall the 9×9 math facts the faster they can perform large number calculations. There are some great math fact tools on rightlobemath.com.
The first step in multiplication is knowing which rod of the abacus to begin your calculation. Once you pick a unit rod, the 1’s place, on the abacus the starting rod is the number of rods to the left of the unit rod equal to the sum of the number of multiplicand and multiplier digits. For example if I had the problem 4235 x 7, the starting rod would be 5 rods to the left of the chosen unit rod on the abacus.
To multiply on the abacus we simply compare the multiplicand and multiplier digit by digit recalling our single digit multiplication math facts. We add each multiplication fact to the abacus working from the starting rod on the left toward the unit rod. So multiplication is nothing more than adding a series of multiplication facts.
The only knew math concept is for students to manage place value while doing the multiplication calculation. Place value is easily managed since students enter the multiplication facts always as a 2 digit number. If you have a fact like 6×4=24 you would simply enter 24. But if you had a fact like 5×1=5 you would enter the fact as 05, i.e. thinking 2 digits. This simple mechanism ensures each fact is entered exactly in the correct place value during the calculation.
Let’s take a look at the following example.
258 x 9 = 2,322
To start our problem we first determine how many rods to the left of the unit rod is the starting rod. For this example it will be 4 rods since the multiplicand has 3 digits and the multiplier has 1. So choosing a unit rod we count 4 rods to the left counting the unit rod as 1. To begin our problem we multiply the 2 in 258 by 9 or 2×9=18. We place the first product 2×9=18 starting on the 4th rod to the left of the unit rod as shown below. With respect to our chosen unit rod the interim sum is 1,800.
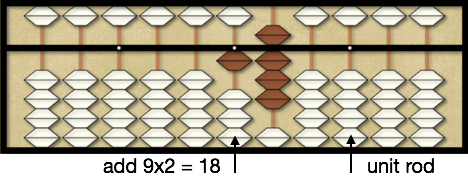
Now we move 1 digit to the right on the multiplicand and also 1 rod to the right on the abacus, the 3rd rod left of the unit rod. Next we place the product of 5×9=45 on the 3rd rod by first adding 4 to 8 on the 3rd rod using a 10 pair addition. So add 1 to the 4th rod and subtract 6, the 10 pair of 4, from 8 on the 3rd rod. Then add the 5 of 45 to 0 on the 2nd rod for an interim sum of 2, 250.
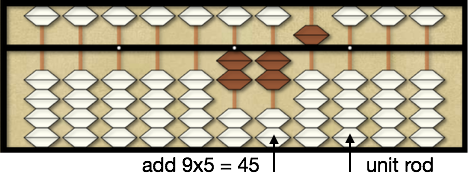
To complete the multiplication we move 1 more digit to the right in the multiplicand and 1 more rod right to the 2nd rod left from the unit rod on the abacus. Now we add the product 8×9=72 to 2, 250 from the 2nd rod. Add 7 to 5 on the second rod using a 10 pair addition. First add 1 to 2 on the 3rd rod and subtract 3, the 10 pair of 7, from 5 on the second rod using a 5 pair by adding 2 and subtracting 5. Lastly add the 2 of 72 to 0 on the unit rod for a final answer of 2,322.
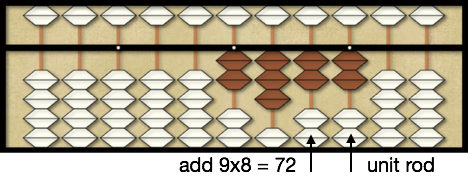
As you can see from the this example how simple, fast, and efficient multiplication is done on the abacus. Now let’s take a look at what happens if my multiplier is more than a single digit number, Multiplication with MultiDigit Multiplier.